1.2 Limits Analyticallyap Calculus
Answer
- 1.2 Limits Analyticallyap Calculus Frq
- 1.2 Limits Analyticallyap Calculus 2nd Edition
- 1.2 Limits Analyticallyap Calculus Calculator
$limlimits f(x)_{x to c}$ exists for all values of c except for at $c=4$
1.2 Limits Analyticallyap Calculus Frq
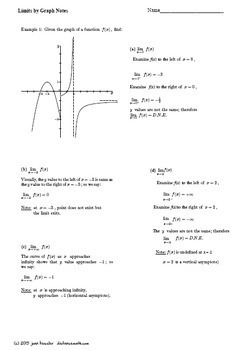
Math AP®︎/College Calculus AB Limits and continuity., that is my x-axis. So defined this way, f of x is equal to x plus 3. So if this is 1, 2, 3, we have. Your AP Calculus students will evaluate limits analytically, including substitution, cancellation, rationalization, and Squeeze Theorem. Your students will have guided notes, homework, and a content quiz on Finding Limits Analytically that cover the concepts in depth from the eight-lesson unit on Limits & Continuity.
1.2 Limits Analyticallyap Calculus 2nd Edition
Work Step by Step
1.2 Limits Analyticallyap Calculus Calculator
A limit is the $y$ value of a function as it gets infinitely close to an $x$ value. A limit exists if $lim limits f(x)_{xto c^{-}}$ $=$ $lim limits f(x)_{xto c^{+}}$. When finding limits on a piecewise function, you first check to make sure each individual 'piece' exists at the given bounds. Then you make sure that at the endpoints of each boundary, the two functions equal each other. In this problem, $limlimits f(x)_{a to 2^{-}}=4$ and $limlimits f(x)_{a to 2^{+}}=4$. However, at the other boundary $x=4$, $lim limits f(x)_{xto 4^{-}}= 0$ and $lim limits f(x)_{xto 4^{+}}=4$, so the limit does not exist at $x=4$
